Hi guys,
can anyone explain it? It’s from SchweserNotes Book 2, Capital Market Expectations.
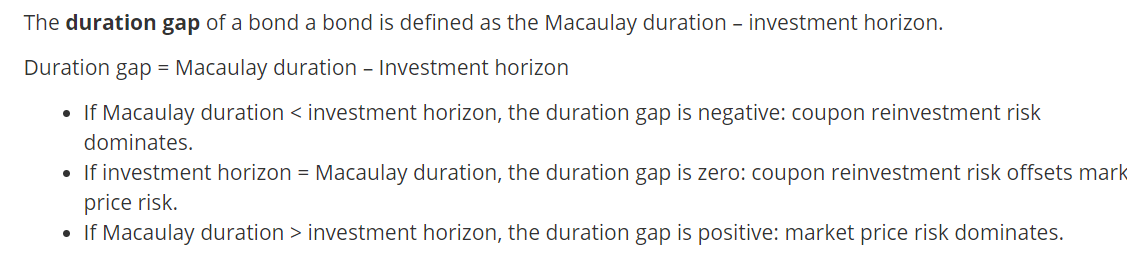
“The overall gain or loss to the investor will depend on the investment horizon. For an investment horizon that is shorter than the Macaulay duration, the capital gain/loss impact will be more dominant than the reinvestment impact, meaning for example that falling (rising) interest rates will result in a higher (lower) realized return. For an investment horizon longer than the Macaulay duration, the reinvestment risk dominates, meaning that falling (rising) interest rates will result in a lower (higher) realized return.”
P.S. I got my ebooks like 2 months ago. Is it true that Book 2 contains bunch of mistakes?
Thanks a lot!
Thanks a lot for your effort. I know this always. But I find it really hard to grasp the essence, WHY is that so.
Look the investment as a cash flow… the two main components (if not all) are interest and principal.
In case of a bond is coupon and face value
In case of a stock is dividend and price
In case of a real estate is rent and market price
etc
If your investment horizon is 5 years and your investment duration is 3 years, then you will have strong risk of reinvestment because as long as your intention is to invest that money for 5 year and the investment will be paid back in just 3 years, you have 2 years the money will be iddle if not find another opportunity.
If your investment horizon is 3 years and your investment duration is 5 years, then you will have strong price risk. Your investment will pay off in 5 years, but you want / have to liquidate that position in year 3, so, your investment may be in a moment of downside by market conditions and loose money. Price risk!
The idea is to have 0 gap between duration and investment horizon. This is a major rule for ALM on banks for example.