I don’t get to catch the idea:
“The convexity of a callable bond turns negative when the call option is near the money, because the upside for the bond is much smaller than the downside (because the value is capped at the call price.) The convexity of a putable bond is always positive because when the option is near the money, the upside for the bond is much larger than the downside (because the floor value is the put price)”
Any help would be appreciated.
Thanks!
With a callable bond
callable bond= stright - call option
putable bond= stright + put option.
When the call option is near money, the convexity becomes negative because there’s limited upside, and the call option reduces the bond price, or returns.
but the downside isn’t floored for callable bonds,
For putable bonds, the put option is added on so the convexity is always positive.
Something like that.
S2000magician has a site with great explanations: http://financialexamhelp123.com/convexity/
Here is the quote from the link:
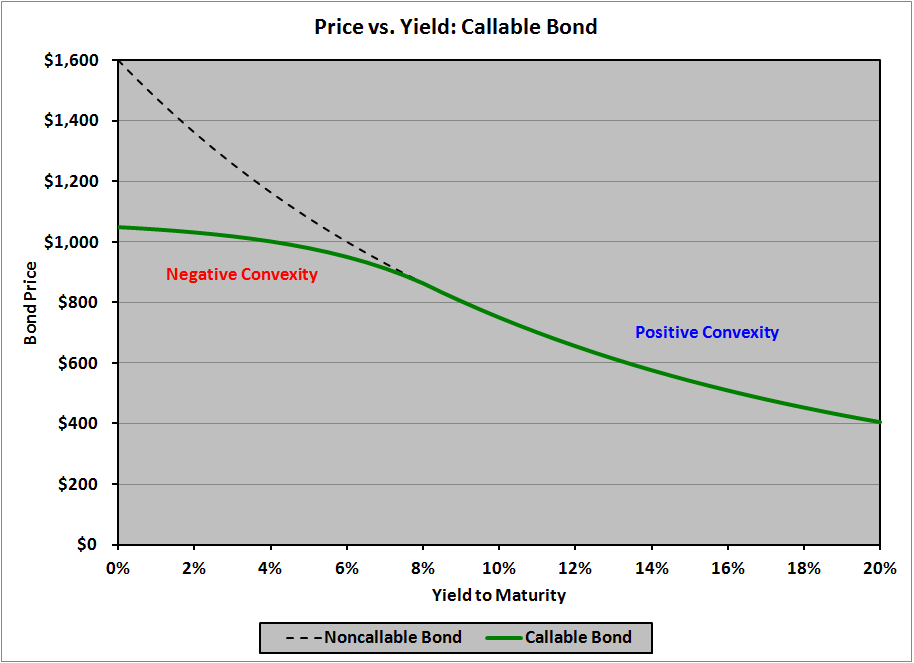
Normally, as a bond’s YTM decreases, not only does the bond’s price increase, but it increases at an increasing rate; i.e., as you move to the left on the price/yield curve, it curves upward. This illustrates positive convexity. When a bond has a call option or a prepayment option, however, there will be a point on the price/yield curve where it will start to curve downward; the price will continue to increase as the YTM decreases, but at a decreasing rate. This illustrates negative convexity. The price/yield graph for a callable bond will look something like this:
1 Like
